is cosecant odd or even|is tangent even or odd : Bacolod All trigonometric functions have parity (either odd or even) and mirror symmetry: Guardians of the Galaxy Volume 3 Science Fiction Adventure Action Peter Quill, still reeling from the loss of Gamora, must rally his team around him to defend the universe along with protecting one of their own.
is cosecant odd or even,Recall that cosine is an even function and sine is an odd function. In terms of equations: $$\cos (-x) = \cos (x)$$. $$\sin (-x) = -\sin (x)$$. We can determine whether each of the other basic trigonometric functions is even, odd, or neither, with just these two facts and .Let us now explore some of the important properties of the cosecant function to understand it better. The graph of cosec x is symmetrical about the x-axis. Cosecant Function is an .Cosine and secant are even; sine, tangent, cosecant, and cotangent are odd. Even and odd properties can be used to evaluate trigonometric functions. See Example .how to determine whether a Trigonometric Function is Even, Odd or Neither, Cosine function, Secant function, Sine function, Cosecant function, Tangent function, and Cotangent function, How to use the .

All trigonometric functions have parity (either odd or even) and mirror symmetry:An odd function is a function f(x) with the property that f( x) = f(x). Examples include x 3 , x 5 , x 7 , sine, and tangent. Cosecant and cotangent are odd functions, meaning that csc( .

The function is an odd function with mirror symmetry: Differentiation. The cosecant is the reciprocal trigonometric ratio of the sine. It is the reciprocal or multiplicative inverse of the sine, that is, csc θ Trigonometric functions are examples of non-polynomial even (in the case of cosine) and odd (in the case of sine and tangent) functions. The properties of even and odd functions are useful in analyzing . y = cos x is always going to be even, because cosine is an even function. For example, cos #pi/4# in the first quadrant is a positive number and cos #-pi/4# (same as cos #pi/4#) in the fourth quadrant is also positive, because cosine is positive in quadrants 1 and 4, so that makes it an even function.(When comparing even and odd function, .
A function is said to be even if \(f(−x)=f(x)\) and odd if \(f(−x)=−f(x)\). Cosine and secant are even; sine, tangent, cosecant, and cotangent are odd. Even and odd properties can be used to evaluate trigonometric functions. See Example. The Pythagorean Identity makes it possible to find a cosine from a sine or a sine from a cosine.In other words, it does not fall under the classification of being even or odd. Examples of How to Determine Algebraically if a Function is Even, Odd, or Neither. Example 1: Determine algebraically whether the given .
is tangent even or oddIn particular, horizontal and vertical shifts can make an odd function even or an even function odd. For example c o s ( x − π 2) maps cosine to sine. Therefore, c o s ( x − π 2) is odd. Transformations can also make it so that a function is neither odd nor even. The transformation s i n ( x) − 1 is an example.
Even and odd functions are categorized according to their symmetry properties. These functions are named because a power function, specifically the nth power of x, is an even function when n is even, and an odd function when n is odd. . Only sine and cosecant have positive values in the second quadrant. Tangent and cotangent are .Determine if Odd, Even, or Neither f(x)=csc(x^2) Step 1. Find . Tap for more steps. Step 1.1. Find by substituting for all occurrence of in . A function is even if . Tap for more steps. Step 2.1. Check if . Step 2.2. Since , the function is even. The function is even. The function is even. Step 3 . This trigonometry video explains how to use even and odd trigonometric identities to evaluate sine, cosine, and tangent trig functions. This video contains .is cosecant odd or even Find exact values of the trigonometric functions secant, cosecant, tangent, and cotangent of π3,π4,π3,π4, and π6.π6. Use reference angles to evaluate the trigonometric functions secant, tangent, and cotangent. Use properties of even and odd trigonometric functions. Recognize and use fundamental identities.
Cosecant function is odd. csc(-x) = - csc x Tangent function is odd. tan(-x) = - tan x Cotangent function is odd. cot(-x) = - cot x. Determine Whether A Trigonometric Function Is Odd, Even, Or Neither. Examples with Trigonometric Functions: Even, Odd or Neither Cosine function, Secant function, Sine function, Cosecant function, Tangent function .For example, if n = 3, we get an even number 6 and an odd number 7. For n = – 5, we get an even number – 10 and an odd number – 9. Properties of Even and Odd Numbers. Take a look at the properties of even and odd numbers, such as addition, multiplication, and subtraction. Property of Addition. Even number + Odd number = Odd numberDetermine if Odd, Even, or Neither f(x)=cos(2x) Step 1. Find . Tap for more steps. Step 1.1. Find by substituting for all occurrence of in . Step 1.2. Multiply by . Step 2. A function is even if . Tap for more steps. Step 2.1. Check if . Step 2.2. Since , the function is even. The function is even. The function is even. Step 3 .
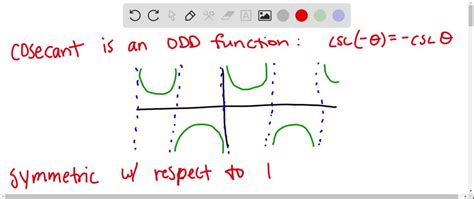
The cosecant graph has vertical asymptotes at each value of \(x\) where the sine graph crosses the \(x\)-axis; we show these in the graph below with dashed vertical lines. Note that, since sine is an odd . A property of odd functions is that they have origin symmetry, which means the graph can is symmetrical when reflected over the point (0,0). Odd. Know that sin (-x)=-sin (x). Even function: when f (-x)=f (x) Odd function: when f (-x)=-f (x) In this case, f (x)=cscx f (x)=1/sinx f (-x)=1/ (sin (-x)) f (-x)=1/ (-sinx) f (-x)=-cscx=-f (x) Thus .Where the graph of the sine function increases, the graph of the cosecant function decreases. The cosecant graph has vertical asymptotes at each value of x where the sine graph crosses the x-axis; we show these in the graph below with dashed vertical lines. Note that, since sine is an odd function, the cosecant function is also an odd function.is cosecant odd or even is tangent even or oddThe cosecant graph has vertical asymptotes at each value of \(x\) where the sine graph crosses the \(x\)-axis; we show these in the graph below with dashed vertical lines. Note that, since sine is an odd function, the cosecant function is also an odd function. That is, \(\csc(−x)=−\csc x\).
Cosine and secant are even; sine, tangent, cosecant, and cotangent are odd. Even and odd properties can be used to evaluate trigonometric functions. See . The Pythagorean Identity makes it possible to find a cosine from a sine or a sine from a cosine.
You can find whether the given number is even or odd in the blink of an eye taking the help of Even or Odd Calculator. The tool is pretty much straight forward and all you need to do is just provide inputs and tap on the enter button to avail the result. Ex: Is 25 even or odd. Is 46 even or odd.Cosine and secant are even; sine, tangent, cosecant, and cotangent are odd. Even and odd properties can be used to evaluate trigonometric functions. The Pythagorean Identity makes it possible to find a cosine from a sine or a sine from a cosine.
is cosecant odd or even|is tangent even or odd
PH0 · is xcosx even or odd
PH1 · is tangent even or odd
PH2 · is secx even or odd
PH3 · is secant even or odd
PH4 · is cotangent even or odd
PH5 · is cosine even or odd
PH6 · how to find cosecant
PH7 · cosecant formula
PH8 · Iba pa